Published articles about flox:
Article Wasserwirtschaft 6/2017 (german)
Article FAN-Agenda about advantages of structured meshs (german)
flox-GPU is tested and validated in regard of numerical stability, robustness, accuracy, dry-wet interfaces and special software features. A selection of standard-tests is presented for this purpose. These tests either compare against exact analytical solutions or field/laboratory measurements.
Test 1: Lake at rest, complex topography
Test 2: Lake at rest, partially saturated cells
Test 3: Dam-break over dry, plane surface (Ritter)
Test 4: Dam-break over wet, plane surface (Stoker)
Test 5: Dam-break on inclined surface (Dressler)
Test 6: Circular dam-break
Test 7: Malpasset dam-break in France (1959)
Test 1: Lake at rest, complex topography
This basic test investigates the well-known problem of unphysical waves or oscillations at a lake at rest in presence of irregular topography. The problem often arises due to an unbalance of the bed source term and the pressure term in the shallow-water equations. So called ‘well-balanced’ schemes handle this test case without generating unphysical fluxes.
Download: test_lake_irregular.zip
Setup:
A lake at rest with constant water level and without sources or outflow is set-up. The bed topography is strongly irregular.
Results:
flox-GPU is ‘well-balanced’ in presence of an irregular topopgraphy: the water level remains at rest, no unphysical fluxes or oscillations are generated and the velocities remain zero.
Test 2: Lake at rest, partially saturated cells
Similar to Test1, this test investigates the model’s ‘well-balancing’ property. Special focus hereby is the presence of partially saturated cells in the domain.
Download: test_lake_bump.zip
Setup:
An inclined surface (bump) is used as mesh which is partially flooded with water. A dry-wet interface is formed to the left and right of the bump.
Results:
The water level remains constant and no fluxes or velocities are generated. flox-GPU is ‘well-balanced’ also in the presence of dry-wet interfaces.
Test 3: Dam-break over dry, plane Surface (Ritter)
Dam break studies and the propagation of dam-break waves are an important engineering task and are suited well to test the model’s robustness and accuracy. Furthermore, this test investigates the model’s handling of wave propagation into dry areas. An analytical solution was derived by Ritter.
Download: test_ritter.zip
Setup:
A water reservoir is set-up with a constant water level of 10 m. The reservoir is separated by a dam from a completely dry area to the right. The dam is removed instantaneously at time t=0.0 s, leading to a wave formation. The flow is frictionless.
Results:
Comparison with analytic solution:
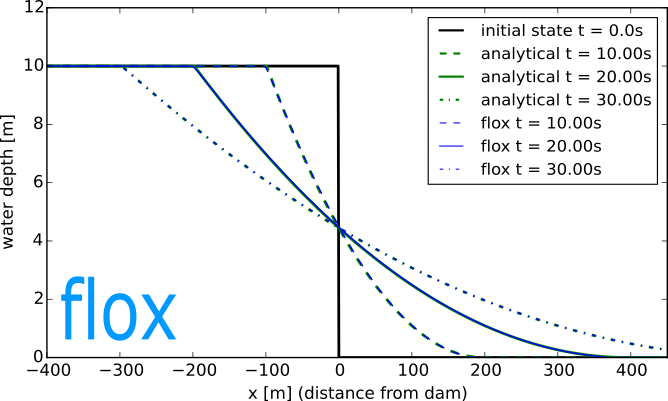
The numerical results fit the analytical solution excellently. The flow characteristics are reproduced with high accuracy :
- The front wave propagation to the right
- The wetting of initially dry elements
- The backward rarefaction wave into the reservoir to the left
- The rotation of the water level around the initial dam location
- The parabolic water level
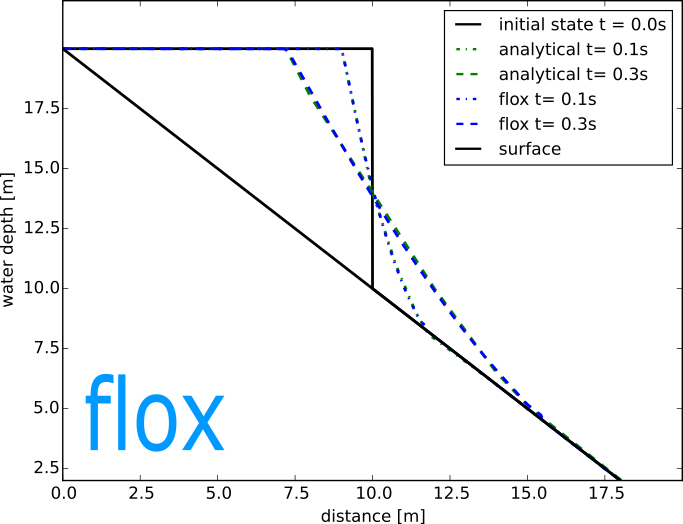
Test 4: Dam-break over wet, plane Surface (Stoker)
This test case is similar to the Ritter-test, but here the water is released into an initially wet area. Hence, a shock wave forms, with a vertical water front, propagating to the right without changing its shape. This test checks the model’s capability to handle shock-waves and discontinuities in the conserved variables. An analytical solution was derived by Stoker.
Download: test_stoker.zip
Setup:
A water reservoir is set-up with a constant water level of 10 m. This reservoir is separated by a dam from another reservoir with a shallow water level of 1 m to the right. The dam is removed instantaneously at time t=0.0 s. The flow is frictionless.
Results:
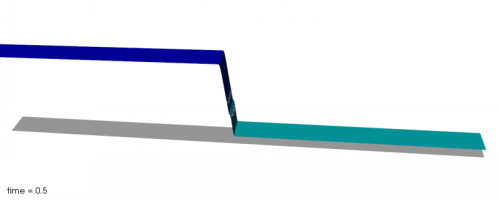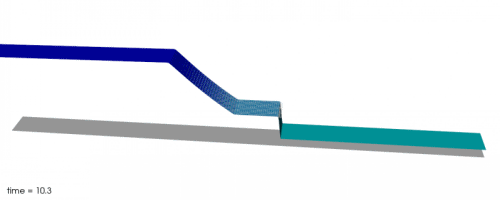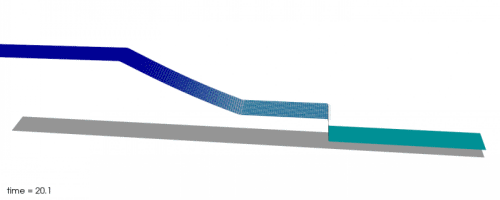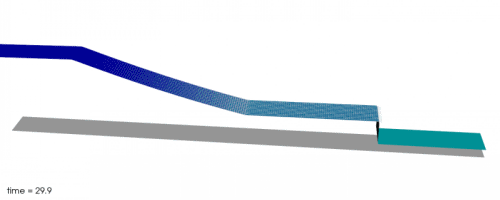Comparison with analytic solution:
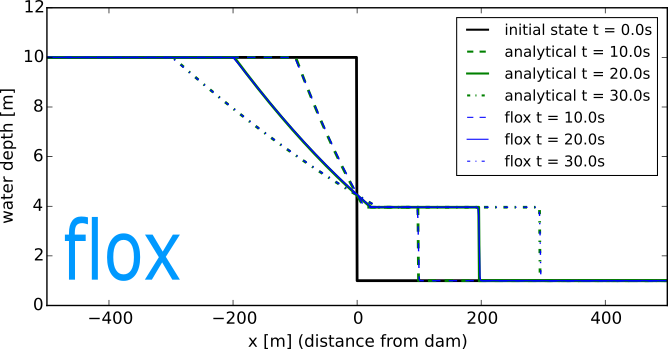The numerical results fits the analytical solution excellently. The flow characteristics are reproduced with high accuracy :
- The shock wave is formed and propagates to the right
- No oscillations are generated at the shock-wave and the front remains sharp without smearing or artificial diffusion
- The backward rarefaction wave into the reservoir to the left
- The rotation of the water level around the initial dam location
Test 5: Dam-break on inclined surface (Dressler)
A water reservoir is released on an inclined, steep surface with 45° slope. The wave front and propagation are stretched by the gravity source term in the shallow water equations. A semi-analytical solution was derived by Dressler.
Download: test_dressler.zip
Setup:
A water reservoir is set-up with initially constant water level of 20 m on a plane with 45° inclination. The reservoir is separated by a dam from a dry area to the right. The dam is removed instantaneously at time t=0.0 s. The flow is frictionless.
Results:
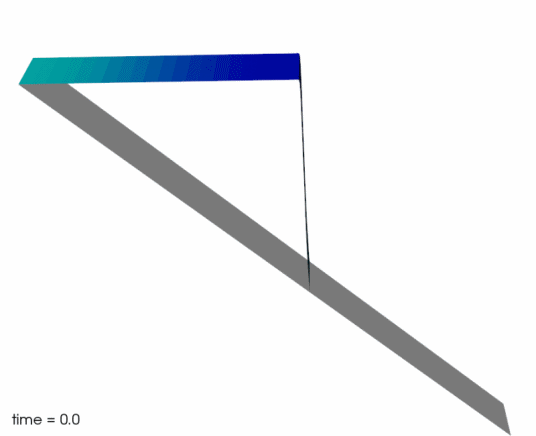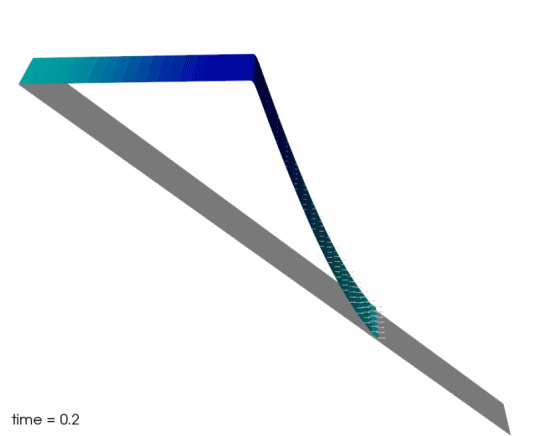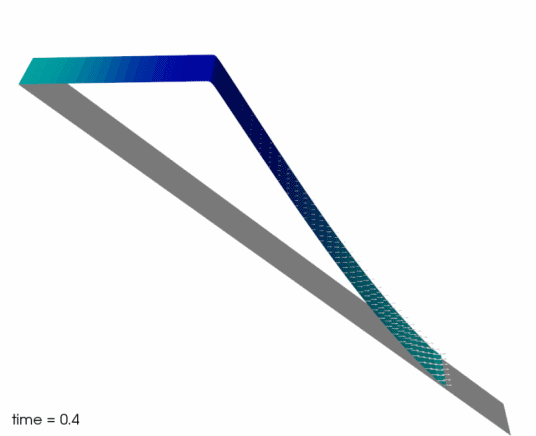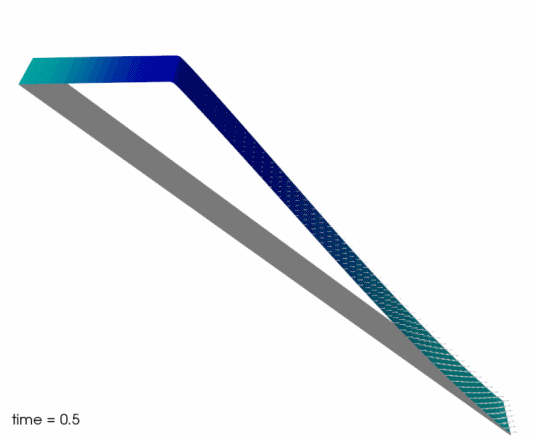Comparison with analytic solution:
The numerical results fit the analytical solution very well.
- The shock wave is formed and propagates to the right. The wave is stretched by the acting gravity force and the wave front is accelerated
- The backward rarefaction wave into the reservoir to the left
- The rotation of the water level around the initial dam location
note: at such steep slopes (45°) 3D effects occur in reality, which are not captured by the shallow-water equations.
Test 6: Circular dam-break
The circuclar dam-break test investigates the spatial formation of circular dam-break and rarefaction waves. The model shall preserve the circular orientation of the wave structures and reproduce the complex flow characteristics.
Download: test_circular_dam.zip
Setup:
A cylindrical water column of 2.5 m height is placed at the centre of the domain. The water column is released at t=0.0 s into the surrounding wet domain with 0.5 m water depth. The flow is frictionless.
Results:
The main flow characteristics are reproduced succesfully (see Toro):
- A circular shock-wave is formed and propagates outwards with a steep front. The wave remains circular during the whole test
- An inward-propagating rarefraction wave is formed
- The inward rarefraction reaches the centre of the domain around t = 0.4 s.
- The inward rarefraction wave finally implodes into the centre and is reflected, producing a fall of the free-surface in the centre. Around t=1.0 s the water level in the centre reaches the initial water depth and falls below this level (overexpansion)
- Due to the overexpansion, a secondary shock-wave is formed and propagates towards the centre
- Around t=5 s the secondary shock-wave implodes at the centre and a sharp characteristic dip is formed.
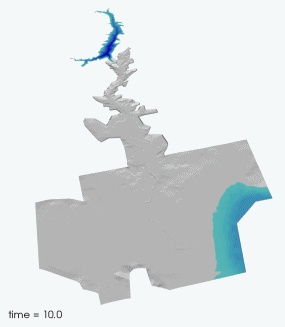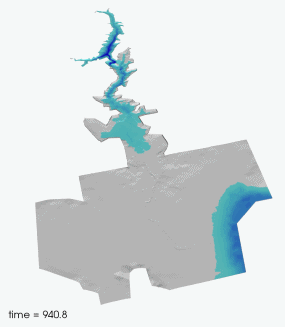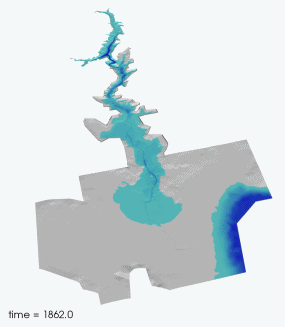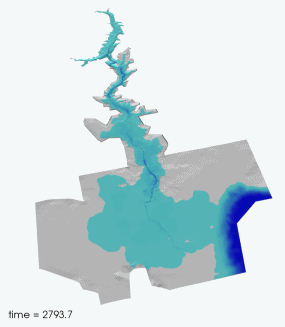
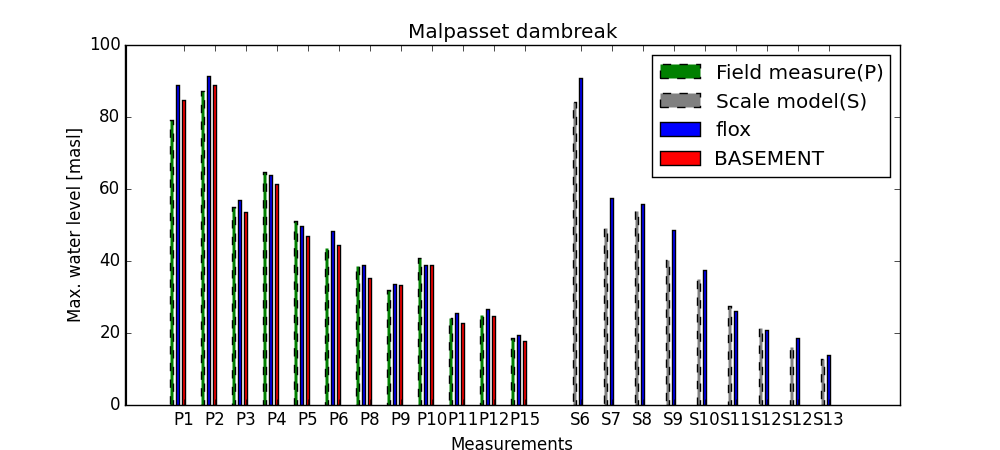
Test 7: Malpasset dam-break in France (1959)
The Malpasset Dam was an arch dam, located at the Côte d’Azur in southern France. It collapsed in 1959, killing 423 people in the resulting flood wave. This real event is often used for model validation, because field measurements and scale-model data are available for comparison. The dam-break wave reached heights of 40 m and very high velocities and inundated large areas (dry-wet transition), making it a challenging test for numerical codes.
Download: test_malpasset.zip
Setup:
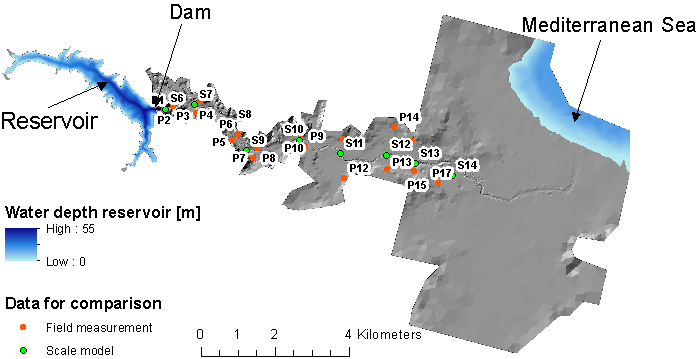The Malpasset reservoir is initially filled to the water level of 100 masl. The dam is removed instantaneously at t=0.0 s and the dam-break wave propagates down the valley. A Strickler roughness value of 25 m¹/³/s is set over the whole domain.
Results:
Comparison with measurements:
The maximum water levels are compared along the downstream valley with the field measurements and the results of the scale model. Overall, flox-GPU reproduces the water levels to a satisfactorily degree without special model calibration. The largest deviations occur in the direct vicinity of the dam, which may be attributed to 3D effects and special aspects (e.g. non-instantaneous, partial dam-break) not captured by the model.